Page 14 - Math Course 3 (Book 2)

Properties of Parallelograms
Mo. 7

Lesson 2

KEY CONCEPTS:

1. Recognize and apply properties of the
sides and angles of parallelograms.
2. Recognize and apply properties of
diagonals of parallelograms.

MO. 7 - L2a

Recognize and Apply the

Properties of Parallelograms

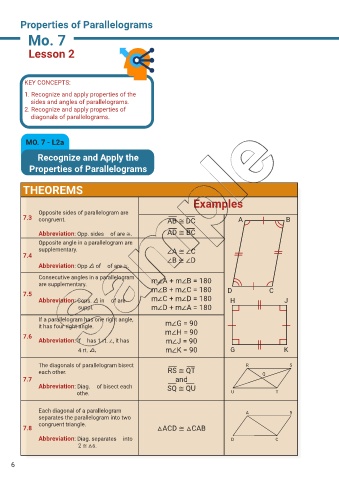
THEOREMS

Examples

Opposite sides of parallelogram are
7.3 congruent. AB ≅ DC A B

AD ≅ BC
Abbreviation: Opp. sides ▱ of are ≅.
Opposite angle in a parallelogram are
supplementary. ∠A ≅ ∠C
7.4 ∠B ≅ ∠D
Abbreviation: Opp ⦞ of ▱ of are ≅.

Consecutive angles in a parallelogram m∠A + m∠B = 180
are supplementary. m∠B + m∠C = 180

7.5 D C
Abbreviation: Cons. ⦞ in ▱ of are m∠C + m∠D = 180 H J
suppl. m∠D + m∠A = 180

If a parallelogram has one right angle, m∠G = 90
it has four right angle.
7.6 m∠H = 90
Abbreviation: If ▱ has 1 rt. ∠, it has m∠J = 90
4 rt. ⦞. m∠K = 90 G K

The diagonals of parallelogram bisect R S
each other. RS ≅ QT Q
7.7 and
Abbreviation: Diag. ▱ of bisect each SQ ≅ QU
othe. U T

Each diagonal of a parallelogram A B
separates the parallelogram into two
congruent triangle.
7.8 △ACD ≅ △CAB
Abbreviation: Diag. separates ▱ into D C
2 ≅ △s.

6